Simple Interest
When a person borrows some amount of money from another person or organisation (bank), then the person borrowing money (borrower) pays some extra money during repayment, that extra money during repayment is called
interest.
For example, If A takes Rs. 50 from B and after using Rs. 50, A returns Rs. 55 to B, then A pays (55-50) i.e., Rs. 5 as interest.
Let us consider following definitions before proceeding exercise
Principal (P) : Principal is the money borrowed or deposited for a certain time.
Interest (I) : Extra money paid for using other's money is called interest.
Amount (A) : The sum of principal and interest is called amount.
Amount = Principal + Simple Interest
Rate of Interest (R) : It is the rate at which the interest is changed on principal. It is always specified in percentage terms
Time (T) : The period, for which the money is borrowed or deposited, is called time.
Basic Formula Related to Simple Interest
Simple Interest (S.I.):
If the interest is calculated on the original principal for any length of time, then it is called simple interest.
Let Principal = P, Rate = R% per annum (p.a.) and Time = T years. Then
| (i). Simple Intereest = |
 |
P x R x T |
 |
| 100 |
| (ii). Principal(P) = |
 |
100 x S.I. |
 |
| R x T |
| (iii). Rate(R) = |
 |
100 x S.I. |
 |
| P x T |
| (iv). Time(T) = |
 |
100 x S.I. |
 |
| P x R |
TIPS on cracking Aptitude Questions on Simple Interest
Tip #1:Understand the formulae
1. Amount to be repaid after N years if simple interest is applied = P + (P x N x R) = P (1 + N x R)
2. Simple Interest = P x N x R
3. Amount to be repaid after N years if interest is compounded = P [(1 + R)^N]
4. Compound Interest = [P x (1 + R)^N] - P
Question: Simple interest on a certain sum of money for 3 years at 8% per annum is half the compound interest on Rs.4000 for 2 years at 10% per annum. Calculate the principal placed on simple interest.
Solution:
Let the Principal be Rs. P
Then, SI = (P x R x T) = 0.24P
Given CI = 4000(1 + 0.1)2 – 4000 = 4000(1.21 – 1) =4000 x 0.21
According to the question,
0.24P = 2000 x 0.21
=> P = 2000 x 0.21 / 0.24 = 2000 x 7 / 8 = Rs. 1750
Tip #2:If the interest rate is applied on a half-yearly, quarterly or monthly basis, the effective annual rate is calculated by compounding the interest
Question: What is the effective annual rate of interest corresponding to a nominal rate of 6% per annum payable half-yearly?
Solution:
Let the Principal be Rs. P.
Now, the rate is 6% per annum but the interest has to be paid twice a year (i.e.) an interest of 3% is applied every 6 months.
Amount to be repaid after 1 year = P x 1.03 x 1.03 = 1.0609P
=> Effective Annual Rate of interest = 6.09%
TTip #3:Use logarithms to find the time when compound rates are applied
1. log 2 = 0.301
2. log 3 = 0.477
3. log 4 = 0.602
4. log 5 = 0.699
5. log 6 = 0.778
6. log 7 = 0.845
Question: At 3% annual interest compounded monthly, how long will it take to double your money?
Solution:
Let the number of months be n and the Principal be Rs. P.
Then, P(1 + 0.03)n = 2P
=> (1 + 0.03)n = 2
=> n log ( 1.03) = log 2
=> n = log 2 / log 1.03 = 0.301 / 0.128 = 23.5
Thus. It’ll take 1 year and 11.5 months.
Compound Interest
As we know that when we borrow some money from bank or any person, then we have to pay some extra money at the time of repaying. This extra money is known as interest. If interest accrued on principal, it is known as simple interest.
Sometimes it happens that we repay the borrow money some late. After the completion of specific period, interest accrued on principal as well as interest due of the principal.
Then, it is known as compound interest.
Compound interest = Amount - Principal
Basic Formula Related to Compound Interest
Let Principal = P, Rate = R% per annum, Time = n years.
1. If interest is compound annually, then:
| Amount = P |
 |
1 + |
R |
 |
n |
| 100 |
Compound interest = Amount - Principal
2. If interest is compounded half-yearly, then R = R/2 and n = 2n
| Amount = P |
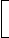 |
1 + |
(R/2) |
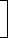 |
2n |
| 100 |
3. If interest is compounded quarterly, then R = R/4 and n = 4n
| Amount = P |
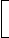 |
1 + |
(R/4) |
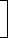 |
4n |
| 100 |
4. If interest is compounded annually but time is in fraction, (Suppose time = 3 years), then :
years), then :
| Amount = P |
 |
1 + |
R |
 |
3 |
x |
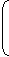 |
1 + |
 R R |
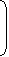 |
| 100 |
100 |
5. When Rates are different for different years, say R1%, R2%, R3% for 1st, 2nd and 3rd year respectively.
| Then, Amount = P |
 |
1 + |
R1 |
 |
 |
1 + |
R2 |
 |
 |
1 + |
R3 |
 |
. |
| 100 |
100 |
100 |
TIPS on cracking Aptitude Questions on Compound Interest
Tip #1:Understand the formulae
1. Amount to be repaid after N years if simple interest is applied = P + (P x N x R) = P (1 + N x R)
2. Simple Interest = P x N x R
3. Amount to be repaid after N years if interest is compounded = P [(1 + R)^N]
4. Compound Interest = [P x (1 + R)^N] - P
Question: Simple interest on a certain sum of money for 3 years at 8% per annum is half the compound interest on Rs.4000 for 2 years at 10% per annum. Calculate the principal placed on simple interest.
Solution:
Let the Principal be Rs. P
Then, SI = (P x R x T) = 0.24P
Given CI = 4000(1 + 0.1)2 – 4000 = 4000(1.21 – 1) =4000 x 0.21
According to the question,
0.24P = 2000 x 0.21
=> P = 2000 x 0.21 / 0.24 = 2000 x 7 / 8 = Rs. 1750
Tip #2:If the interest rate is applied on a half-yearly, quarterly or monthly basis, the effective annual rate is calculated by compounding the interest
Question: What is the effective annual rate of interest corresponding to a nominal rate of 6% per annum payable half-yearly?
Solution:
Let the Principal be Rs. P.
Now, the rate is 6% per annum but the interest has to be paid twice a year (i.e.) an interest of 3% is applied every 6 months.
Amount to be repaid after 1 year = P x 1.03 x 1.03 = 1.0609P
=> Effective Annual Rate of interest = 6.09%
Tip #3:Use logarithms to find the time when compound rates are applied
1. log 2 = 0.301
2. log 3 = 0.477
3. log 4 = 0.602
4. log 5 = 0.699
5. log 6 = 0.778
6. log 7 = 0.845
Question: At 3% annual interest compounded monthly, how long will it take to double your money?
Solution:
Let the number of months be n and the Principal be Rs. P.
Then, P(1 + 0.03)n = 2P
=> (1 + 0.03)n = 2
=> n log ( 1.03) = log 2
=> n = log 2 / log 1.03 = 0.301 / 0.128 = 23.5
Thus. It’ll take 1 year and 11.5 months.